Residual Stress Measurement

What is Residual Stress?
Residual stress refers to the stress that remains in a material after the original cause of the stress has been removed. These stresses can be introduced during manufacturing processes such as casting, welding, machining, or heat treatment. Residual stress can exist in various forms, including tensile, compressive, or shear stresses, and it can influence the material's mechanical properties, performance, and structural integrity. Understanding and managing residual stress is crucial in engineering to ensure the reliability and longevity of components and structures.
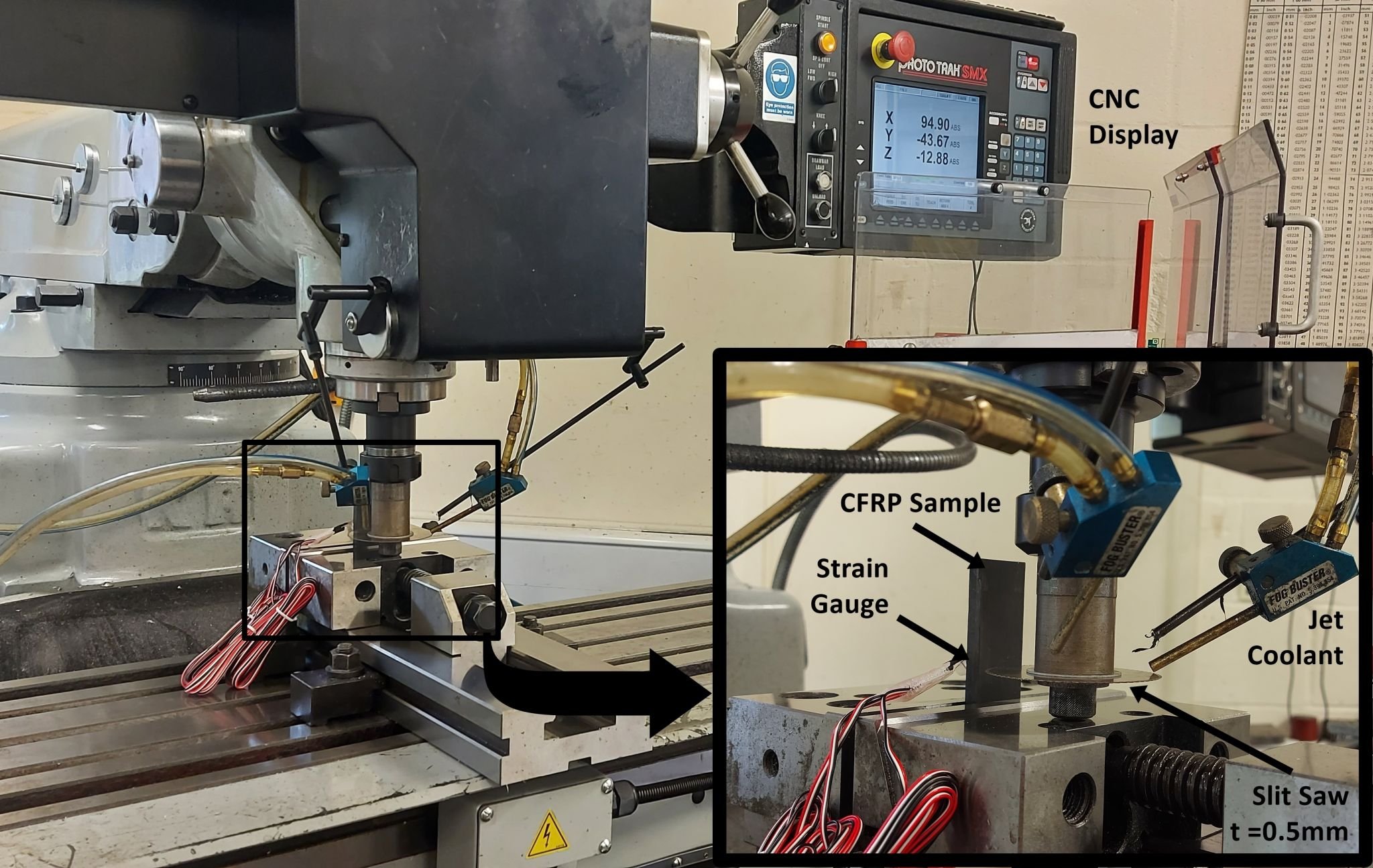
Slitting Experiment
It is one of the strain release technique which involves cutting a slit across a specimen, typically using wire - Electric Discharge Machining, and measuring the surface strains with strain gauges located underneath and top surface of specimen next to the slit as shown in the Figure. During the procedure the slit depth is increased incrementally to predetermined depths and the strain at each depth increment is recorded. In polymer composites, the surface strain relaxations are related to the stresses throughout the specimen thickness using numerically determined influence or crack coefficients. Typically the underneath strain gauge is most important and is used for the calculation of through thickness residual stresses, however the top strain gauge is only reliable for only surface stresses during the first few EDM increments.
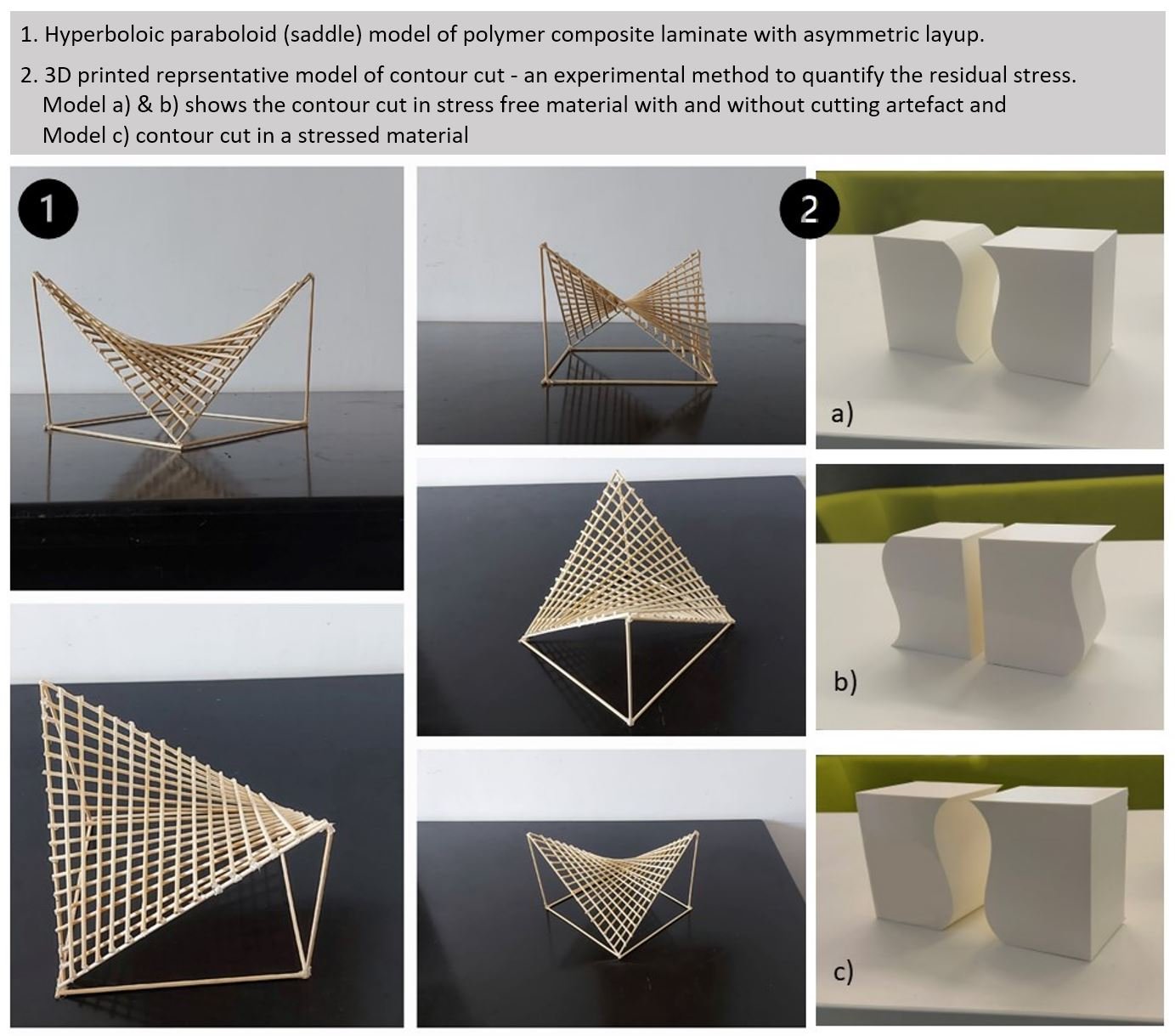
Contour Method
The contour method is based on the application of Bueckner’s linear superposition principle, where the relaxation of residual stresses owing to the cutting process is assumed to be linear elastic. The notion forms the cornerstone for using the material's linear-elastic behaviour to implement the contour approach. The superposition principle plays as an invaluable concept in Fracture Mechanics and can be extended to many applications. It states –
“Any elastic crack or notch problem can be reduced to one where the external load appears in the form of tractions distributed over the faces of the crack” [1]. The essential condition in this statement is that the material must be elastic. Such material having stress variation experiences elastic displacement on its cut surfaces upon severing into half due to release of residual stress. Applying these displacement profile as surface traction onto the cut surface in Finite Element Analysis it is possible to calculate the initial residual stress field that was present in the material as illustrated in the Figure.
[1] Bueckner HF. The Propagation of Cracks and the Energy of Elastic Deformation. J Fluids Eng. 1958;80(6):1225–9.
Classical Laminate Theory (CLT) is a simple analytical method to assess laminated composites based on the concept of standard plate theory proposed by Kirchhoff- Love [1]. The theory is an extension of Euler-Bernoulli’s beam theory developed by Love using the assumptions of Kirchhoff. The analytical model has been successfully applied on laminated polymer composites [2,3.4.5] and had good prediction against experimental values [6,7].
The behaviour of the Laminate is predicted under the framework of the following assumptions,
Each ply is regarded as homogeneous and its effective properties are known.
The behaviour of each ply can be isotropic, orthotropic or transversely isotropic orthotropic.
Each ply is under state of plane stress.
The strain across the laminate thickness is continuous as shown in the Figure 2- 25. In other words, each ply in a laminate is perfectly bonded and no slip is observed between them.
Straight lines perpendicular to the mid-surface (imaginary surface at the middle of layup sequence of the laminate) remain straight even after the deformation.
Straight lines perpendicular to the mid-surface remain perpendicular even after the deformation.
The thickness of the laminate remains unaltered throughout the deformation.
The last three assumptions are similar to the assumptions in Euler-Bernoulli’s beam theory. Based on these assumptions the stresses in the laminate at macroscopic level can be evaluated. Similarly, the macroscopic residual stress generated during fabrication can be calculated using curing temperature range. The following elements, however, that impact residual stress are ignored:
Viscoelastic behaviour and chemical shrinkage of resin and transversely isotropic behaviour of the fibre are not considered in CLT.
Stress-relaxation is ignored from the viscoelastic behaviour of the epoxy [8,9].
The cure temperature dependence of the composite properties, especially CTE, is neglected in the CLT.
The cooling rate can affect the values of residual stresses. Slower cooling rate could result in lower residual stresses.
Classical Laminate Theory
REFERENCES
[1] A. E. H. Love. Love Plate Theory. Philos Trans R Soc Lond [Internet]. 1888 [cited 2023 Mar 25];A, 179(1888):491–546. Available from: https://shellbuckling.com/papers/1888love.pdf
[2] Staab GH. Laminar Composites. In: Second Edition. Butterworth-Heinemann; 2015. p. 189–284.
[3] Shokreih MM. Residual Stress in Composite Materials. 2014.
[4] Ghasemi AR, Tabatabaeian A. A unified comparative study on the classical lamination theory, hole drilling, slitting, and curvature measurement methods for assessment of residual stress in laminated polymeric composites. Mechanics of Materials. 2022 Apr 1;167.
[5] Sabaté N, Vogel D, Gollhardt A, Keller J, Cané C, Gràcia I, Morante JR, Michel B. Measurement of residual stress by slot milling with focused ion-beam equipment. Journal of Micromechanics and Microengineering [Internet]. 2006 Jan;16(2):254. Available from: https://dx.doi.org/10.1088/0960-1317/16/2/009
[6] Hahn HT, Pagano NJ. Curing Stresses in Composite Laminates. J Compos Mater [Internet]. 1975;9(1):91–106. Available from: https://doi.org/10.1177/002199837500900110
[7] Hahn HT. Residual Stresses in Polymer Matrix Composite Laminates. J Compos Mater [Internet]. 1976;10(4):266–78. Available from: https://doi.org/10.1177/002199837601000401
[8] Zhao LG, Warrior NA, Long AC. A. Thermo–viscoelastic analysis of process induced residual stress in fibre-reinforced polymer–matrix composites. Mater Sci Eng A. 2007;22:483–98.
[9] Zhang Y, Xia Z, F. E. Evolution and influence of residual stresses/strains of fiber reinforced laminates. Compos Sci Technol. 2004;64(10-11):1613–21.